Have you ever wondered how you can calculate the volume of various geometric shapes accurately and effortlessly? With the help of volume calculators, this task becomes straightforward, even when dealing with complex figures. These tools not only simplify mathematical calculations but also provide a practical way to understand and apply the concept of volume in everyday and professional contexts.
 Volume Calculator
Volume Calculator

This image is property of www.calculator.io.
Volume Calculator Features
Volume calculators come equipped with a variety of features designed to make your calculations as seamless as possible. Let’s check out some of these capabilities.
Performs Calculations for 11 Geometric Shapes
Volume calculators can handle calculations for a diverse range of geometric shapes. These include basic forms like spheres, cones, and cubes, as well as more complex shapes such as capsulated and frustum figures. This allows for a wide range of applications, whether you are a student, an engineer, or someone in a scientific field.
Supports a Variety of Units of Measurement
Accuracy in calculations is crucial, and often you need to switch between different units of measurement. Volume calculators support both metric and imperial units, making it convenient to convert from meters to feet or from centimeters to inches without any hassle.
Demonstrates Solution Steps
One of the most advantageous features of volume calculators is their ability to show the steps involved in the calculations. This helps in understanding the process and ensures transparency in how the final result is derived. Whether you are a learner who is grasping the nuances of geometry or a professional requiring precision, this feature proves immensely useful.
Shapes Supported
Understanding the types of shapes supported by volume calculators helps you see just how versatile these tools can be. Here’s a breakdown of the shapes that can be calculated.
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, much like a basketball. The formula to calculate its volume is: [ V_ = \fracπ r^3 ]
Cone
A cone has a circular base and narrows smoothly to a point called the apex. Its volume is calculated using: [ V_ = \frac π r^2 h ]
Cube
A cube is a three-dimensional square where all sides are equal. The volume of a cube is: [ V_ = a^3 ] where ( a ) is the length of one side.
Cylinder
A cylinder has straight parallel sides and a circular cross-section. To find its volume, use: [ V_ = π r^2 h ]
Rectangular Tank
Commonly found in fish tanks and storage containers, the volume formula is: [ V_ = l × w × h ] where ( l ) is length, ( w ) is width, and ( h ) is height.
Capsule
A capsule consists of a cylindrical body with hemispherical ends. Its volume is: [ V_ = π r^2 h + \fracπ r^3 ]
Spherical Cap
A spherical cap represents a portion of a sphere cut by a plane. Calculating its volume involves complex integrations but can be simplified with a volume calculator.
Conical Frustum
A conical frustum is a portion of a cone that lies between two parallel planes cutting it. Its volume can be calculated using appropriate formulas which a volume calculator can handle easily.
Ellipsoid
An ellipsoid is an elongated sphere-like shape defined by three principal axes. Its volume calculation formula is more complex, but manageable through a volume calculator.
Square Pyramid
A square pyramid has a square base and four triangular faces converging to a single point (the apex). The formula to determine its volume is: [ V_ = \frac l w h ]
Tube
A tube is essentially a hollow cylinder. To compute its volume, you measure the dimensions of the outer and inner cylinders and find the difference.
Units Supported
When performing volume calculations, you may need to toggle between different units of measurement to ensure precision and applicability in various contexts. Here are the units supported:
Metric Units
Metric units are widely used globally and include:
- Meters (m)
- Kilometers (km)
- Centimeters (cm)
- Millimeters (mm)
- Micrometers (µm)
- Nanometers (nm)
- Angstroms (Å)
Imperial Units
Imperial units are commonly used in the United States and include:
- Miles (mi)
- Yards (yd)
- Feet (ft)
- Inches (in)
Volume calculators often have built-in converters that make transitioning between these units intuitive and straightforward.
Usage of Volume Calculation
Volume calculation is not just academic; it has a wide range of practical applications in various disciplines.
Important in Various Science and Mathematics Disciplines
The concept of volume is fundamental in fields like physics, chemistry, engineering, and environmental science. Whether you’re calculating the volume of a liquid for a chemical reaction or determining the space within a container, accurate volume measurements are indispensable.
Indicates Space Occupied by an Object or Its Capacity
Understanding the volume of an object helps you determine how much space it occupies and its capacity. For example, architects and builders rely on volume calculations for constructing reservoirs and storage units.

This image is property of www.calculator.io.
Uniformity in Calculations
It’s crucial to ensure uniformity when performing volume calculations to avoid errors.
Conversion to a Consistent Unit Required for Accurate Volume Calculation
When dealing with different units, consistency is key. Convert all measurements to the same unit before performing volume calculations to ensure accuracy.
The Calculator Assists with Unit Conversions and Demonstrates Steps
One of the most powerful features of volume calculators is their ability to assist with unit conversions seamlessly, providing a step-by-step process that enhances understanding and accuracy.
Examples of Volume Calculations
Let’s look at the specific formulas used to calculate the volume of various shapes.
Sphere
[ V_ = \fracπ r^3 ]
Cone
[ V_ = \frac π r^2 h ]
Cube
[ V_ = a^3 ]
Cylinder
[ V_ = π r^2 h ]
Rectangular Tank
[ V_ = l × w × h ]
Capsule
[ V_ = π r^2 h + \fracπ r^3 ]
Using these formulas, volume calculators can quickly provide accurate results, saving you laborious manual calculations.

This image is property of www.calculator.io.
Complex Geometric Shapes
Volume calculators shine when it comes to complex geometric shapes, often combining multiple simpler shapes to derive precise volumes.
Combines Basic Shapes to Calculate Volume
For intricate shapes, volume calculators may break them down into simpler components. For example, a truncated cone could be divided into a smaller cone and a larger cone; their volumes are calculated separately and then added.
Uses Formulas Like Combining Cylinder and Cone Volumes
By combining different geometric shapes like cylinders and cones, you can calculate volumes that would otherwise seem daunting.
Practical Applications
Volume calculations have numerous practical applications, impacting various fields from construction to pharmaceuticals.
Pharmaceutical Scientists Use Capsule Volume Calculations for Medication Storage
Pharmaceutical industries often calculate the volume of capsules to determine accurate storage and dosage requirements. This involves using specific formulas adapted for capsule shapes.

This image is property of www.calculator.io.
Capsule Volume
Capsules are commonly used in pharmaceuticals and require precise volume calculations for effective formulation and storage.
Formula for Capsule Volume
[ V_ = π r^2 h + \fracπ r^3 ]
Having a volume calculator simplifies this process, ensuring precision and efficiency.
Spherical Cap
A spherical cap is a mathematical term for a portion of a sphere, sliced by a plane, and essentially represents a ‘cap’ or ‘hat’ on a sphere.
Represents a Portion of a Sphere Cut by a Plane
Calculating the volume of a spherical cap can be tricky, involving more advanced mathematical techniques. However, a volume calculator can simplify this process by applying the appropriate formulas and presenting clear, step-by-step solutions.
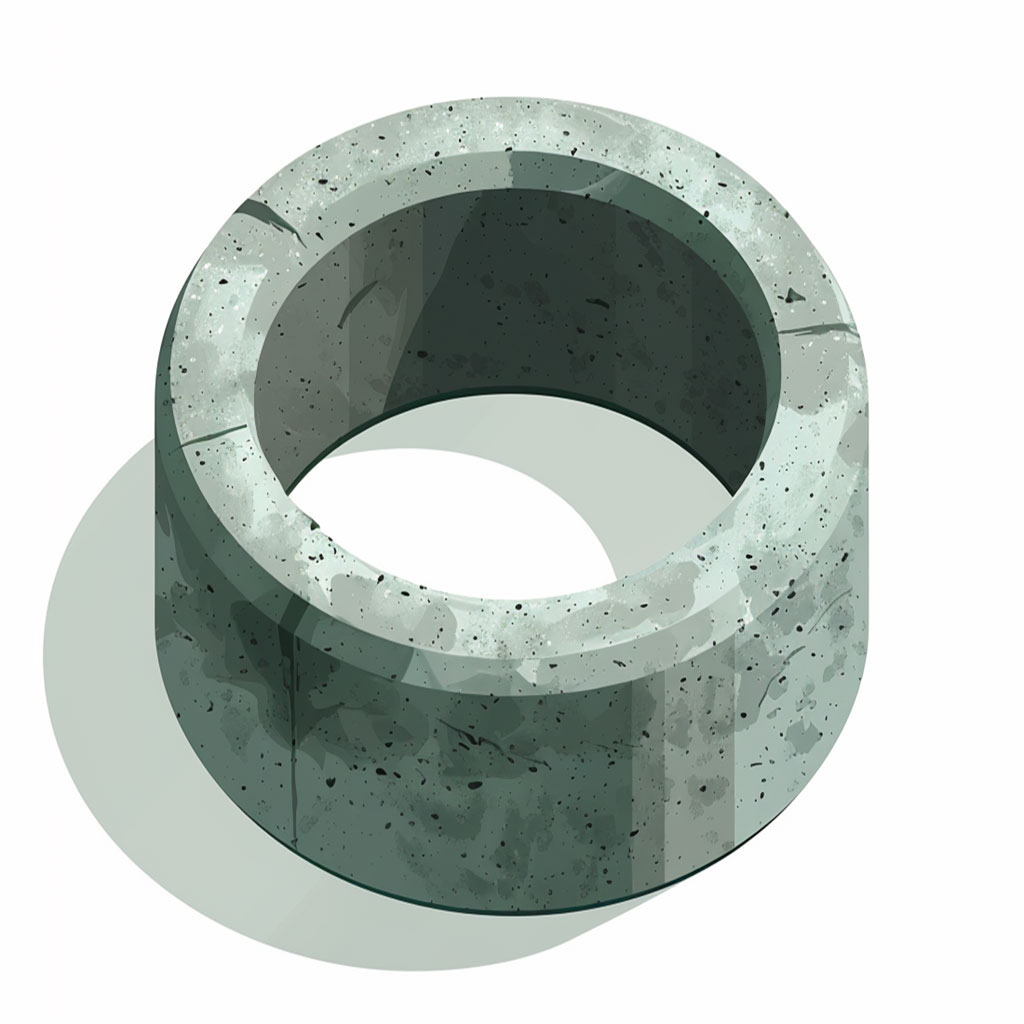
This image is property of www.calculator.io.
Conclusion
Volume calculators are highly versatile tools designed to simplify and streamline the process of calculating the volume of various geometric shapes. From supporting a variety of shapes and units of measurement to demonstrating the calculation steps, these tools make complex calculations easier and more accessible. They serve crucial roles in education, science, engineering, and several other fields, ensuring accuracy and uniformity in volume measurement.
Whether you’re a student trying to understand your geometry homework, an architect working on a new project, or a scientist analyzing experimental data, a volume calculator is an invaluable tool to have in your arsenal. Utilize it to save time, ensure accuracy, and understand the fundamental concepts of volume calculations. Happy calculating!